このブログは、旧・はてなダイアリー「檜山正幸のキマイラ飼育記 メモ編」（http://d.hatena.ne.jp/m-hiyama-memo/）のデータを移行・保存したものであり、今後（2019年1月以降）更新の予定はありません。

今後の更新は、新しいブログ http://m-hiyama-memo.hatenablog.com/ で行います。

多様体の定義

幾何っぽい CADG

比較的標準的

プレ多様体の定義

Let α ∈ N ∪ {∞}. A locally R-ringed space (X, OX) is called (real) Cα-premanifold if there exists an open covering X = ∪(i∈I | Ui) such that for all i ∈ I there exist n ∈ N, an open subspace Y of Rn, and an isomorphism of locally R-ringed spaces (Ui, OX|Ui) ∼→ (Yi, CαY) (called chart). Here Y and n depend on i.

In this case the structure sheaf is denoted by CαX, and is called the sheaf of Cα-functions on X.

A family of charts Φi: (Ui, OX|Ui) ∼→ (Yi, CαYi) is called atlas if ∪(i∈I | Ui) = X. We call a real C∞-premanifold a smooth premanifold.

次の概念が使われている。

  1. open covering
  2. openset Y of Rn
  3. local isomorphism
  4. chart
  5. atlas

多様体の定義

α-manifold (resp. a complex manifold) is a Cα-premanifold (resp. a complex premanifold) whose underlying topological space is Hausdorff and second countable.

プレ多様体が多様体になるのは、台（下部構造）位相空間に対する分離性と可算性の条件が付くだけで、上部構造はプレ多様体とまったく同じ。上部構造は、環付き空間としての構造層と被覆（アトラス）を使って定義する。

あくまで多様体の定義であって、多様体の圏全体を公理的に定義しているわけではない。

Mallios ADGの微分三つ組

Let X be a topological space. A differential triad over X is a triplet δ = (A, ∂, Ω) where A is a sheaf of algebras over X, Ω is an A-module and ∂ : A → Ω is a Leibniz morphism.

Untwisting the definition we have the following data.

∂ : A → Ω is a sheaf morphism such that

  1. ∂ is a C-linear morphism relative to the associated C-vector sheaves A, Ω on X,
  2. ∂ satisfies the Leibniz condition
    ∂(x.y) = x.∂(y) + y.∂(x),
    for any x, y ∈ A ×X A; in other words, for any local sections x, y ∈ A(U) for any U ⊆ X open

これも構造層Aが基本。Aが"代数"だと言っているが、あまりハッキリしない。想定は、Aがなめらかな関数の層で、Ωが1-形式の層。∂は関数の外微分。

構造層の“値”であるA(U)が、標準的（モデル的）開集合Yに対するC∞(Y)と局所同型であることを要求してない。微分概念はライプニッツ射にエンコードしている。ライプニッツ射を持つ代数の概念を層を使って位相空間上に“広げた”もの。

無限次元多様体

無限次元多様体の定義

まずはモデル空間。l.c. は局所凸

A class of l.c. model spaces or l.c. models is a subclass E of the class of real (Hausdorff) locally convex spaces such that E contains the numerical spaces Rn for all n ∈ N.

要するに、有限次元ユークリッド空間を含むような位相ベクトル空間のクラス。位相はハウスドルフで局所凸。多様体の局所モデルとしてふさわしい位相ベクトル空間の類を選んで使う。

多様体の定義はモデル空間のクラスEに対して相対的になる。なので、E-多様体と呼ぶ。極大アトラスを使う。

Let E be a class of l.c. model spaces, and M0 a Hausdorff topological space.

(1) A smooth E-atlas on M0 is a family of pairs A = {(Uα, φα) ; α ∈ A} such that {Uα ; α ∈ A} is an open cover of M0 and for every α ∈ A, there exists a space Eα from the class E and a homeomorphism φα : Uα −→ φα(Uα) ⊂ Eα such that the following compatibility condition is satisfied: for every α, β ∈ A such that Uαβ = Uα ∩Uβ ≠ empty, the transition map φαβ = φα ◦φβ-1 : φβ(Uα ∩ Uβ) −→ φα(Uα ∩ Uβ) is smooth (in the sense of Definition 3.1). [注： smoothは別な所で定義されている]

(2) Given a smooth E-atlas A on M0, a (compatible) chart on M0 is a pair (U, φ) where U is an open subset of M0 and φ is a homeomorphism from U onto an open subset φ(U) of some space from the class E such that the transition map between (U, φ) and every (Uα, φα) ∈ A is smooth.

(3) A smooth E-atlas A is said to be maximal if any chart (U, φ) compatible with A belongs already to A. A maximal atlas is also called a smooth structure, the class E being understood.

Let E be a class of l.c. model spaces. A smooth Emanifold is a pair M = (M0, A) where M0 is a Hausdorff topological space and A is a maximal smooth E-atlas on M0.

チャートとチャート、あるいはチャートとアトラスが整合（compatible, consistent, admissible）するという概念で、極大アトラスを定義できる。

グロタンディーク位相

nLabのグロタンディーク位相の定義：

まず篩

a sieve on an object X in a category C is a collection of morphisms with codomain X that are closed under precomposition with morphisms in C.

[もっと詳しく]

A sieve S on an object c∈C is a subset S⊂Ob(C/c) of the set of objects of the over category over c which is closed under precomposition: it has the property that whenever (d→c)∈S and (e→d)∈Mor(C) then the composition (e→d→c) is in S.

篩の集合を割り当てるグロタンディーク位相

A Grothendieck topology J on a category C is an assignment to each object c∈C c ∈ C of a collection of sieves on c which are called covering sieves, satisfying the following axioms:

  1. If F is a sieve that covers c and g:d→c is any morphism, then the pullback sieve g*F covers d.
  2. The maximal sieve id : hom(−,c) → hom(-, c) is always a covering sieve;
  3. Two sieves F, G of c cover c if and only if their intersection F∩G covers c. (Here the saturation condition is important.)
  4. If F is a sieve on c such that the sieve ⋃d{g:d→c | g*F covers d} is a covering sieve of c, then F itself covers c.

The set of covering sieves of an object c is denoted J(c).

CADGの多様体の作り方

制限圏の定義：

A restriction category is a category X with a combinator, (-)- : X(A, B) −→ X(A, A), satisfying

  • [R.1] f- f = f ; 定義域の部分恒等
  • [R.2] f- g- = g- f- ; 共通部分
  • [R.3] f- g- = (f- g)-;
  • [R.4] f h- = (f h)-f .

A map is total when f = 1.

部分写像の定義域をとる演算の定式化。

多様体の作り方

Manifold completion to tangent structure

(a) From any differential restriction X category we can join complete to obtain a differential join restriction category join(X). Join structure always works well with differentail structure.
(b) Given a differential join restriction category join(X) we can form the manifold completion Man(join(X)).
(c) Man(join(X)) is not a differential restriction category but it has tangent structure .... which axiomatizes the categories arising in differential geometry.

Joinに関して：

In a restriction category, parallel maps f and g are compatible if f- g = g f- .

A restriction category, X, is a join restriction category if every set of compatible maps, C ⊆ X(A, B), has a join (sup) that is stable; i.e.,

  • f(∨(g | g∈C)) = ∨(f g | g∈C)

Theorem: Join and differential restriction structure are compatible; i.e.

  • D[∨(f | f∈C)] = ∨(D[f] | f∈C)

アトラスの定義

If X is a join restriction category, an atlas of objects from X consists of a set of objects Xi ∈ X, together with a series of maps Xi -(φij)→ Xj such that:

  1. φiiφij = φij ;
  2. φijφjk ≤ φik ;
  3. φij has partial inverse φji.

Each map φii : Xi −→ Xi is a restriction idempotent, and represents the “open subset” of Xi that the chart is using. The maps φij define how these charts overlap.

ジョイン制限圏に対して、アトラスの圏Atl(X)を定義できる。アトラスは、PL多様体の三角分割のようなもので、多様体そのものではない。他のアトラスがいくらでも取れる。

Atl(X)をXの多様体の圏と呼ぶのはどうかな？

接圏の定義

(tangent bundles) a cartesian restriction functor X -(T)→ X with a total natural transformation T =(p)⇒ I;

(tangent bundle products) for each M ∈ X and natural number n ≥ 2, the restriction pullback of n copies of TM -(p)−→ M exists and is denoted by (Tn(M), p1 . . . pn), with each pi total: this implies each Tn is a join restriction functor when there are joins, and the arrows Tn -(pi) −−→ T are natural;

(addition of tangent vectors) there are total natural transformations + : T2 −→ T and 0: I −→ T [以下省略、足し算ができること]

(vertical lift) a natural transformation ℓ : T ⇒ T2 such that for each M

  • (ℓM, 0M) : (p : TM→M, +, 0) → (Tp : T2M → TM, T(+), T(0))

is an additive bundle morphism;

(canonical flip) there is a total natural transformation c : T2 ⇒ T2 such that for each M

  • (cM, 1) : (Tp : T2M → TM, T(+), T(0)) → (pT : T2M→TM, +T , 0T )

is an additive bundle morphism;

一貫性条件と普遍性はめんどくさいので画像。

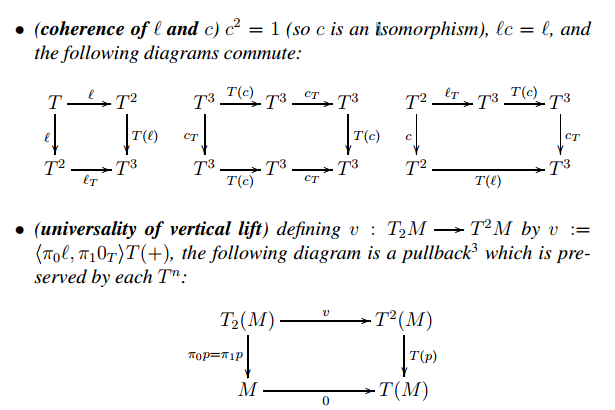
接関手Tを自己関手にしているので、いろいろとめんどくさいことになっている。多様体の圏からベクトルバンドル（加法バンドル）の圏への関手として定義すればよい。自己関手は、バンドルの全空間を取る関手にすればよい。

[追記]
ℓとcの一貫性から次のが分かる。

  • cは T2⇒T2 という対称変換である。
  • cが対称であることは、cc = 1（アルチン／ライデマイスターII） とまんなかの可換図式（アルチン／ライデマイスターIII）が示している。
  • ℓは余対称なセミ余モナドである。セミモナドは単位がないモナドで、セミ余モナド、または余セミモナドはその双対。
  • セミ余モナドの余乗法は対称に対してすり抜けができる（ただし片側だけ）。

接バンドルの足し算構造として、Tの対称モナド構造（ゼロセクションが単位、標準フリップが対称性）が使えないか？と思っている。
[/追記]

拡張デカルト微分圏から接圏を作る：

https://arxiv.org/pdf/1606.08379.pdf から、原文間違っているかも。

  • T(A) := L(A)×A
  • T(f) := <D[f], π1 f>
  • p = π1 射影の定義

AはAの線形台、Dが微分コンビネータ、pはバンドル射影。垂直リフトと標準フリップは次のとおり。

  • ℓ(u, x) = (u, 0, 0, x)
  • c(u, v, w, x) = (u, w, v, x).

[追記]
デカルト微分圏の定義 - 檜山正幸のキマイラ飼育記 メモ編からコピー、そちらに説明あり。

クラットウェル（https://arxiv.org/pdf/1208.4070.pdf）の一般化デカルト微分圏。射の結合は、図式順で併置。射影はπ0とπ1。

A generalized Cartesian differential category consists of a Cartesian category X with:

  • for each object X, a commutative monoid L(X), satisfying
    • L(L(X)) = L(X) and
    • L(X × Y) = L(X) × L(Y),
  • for each map f : X→Y , a map D[f] : L(X)×X →L(Y) such that:
  1. [CD.1] D[+X] = π0 (+X), D[0X] = π0 0X,
  2. [CD.2] 〈a + b, c〉D[f] = 〈a, b〉D[f] + 〈b, c〉D[f] and 〈0, a〉D[f] = 0;
  3. [CD.3] D[π0] = π0 π0, and D[π1] = π0 π1;
  4. [CD.4] D[〈f, g〉] = 〈D[f], D[g]〉;
  5. [CD.5] D[fg] = 〈D[f], π1 f〉D[g];
  6. [CD.6] 〈〈a, 0〉,〈c, d〉〉D[D[f]] = 〈a, d〉D[f];
  7. [CD.7] 〈〈0, b〉,〈c, d〉〉D[D[f]] = 〈〈0, c〉,〈b, d〉〉D[D[f]];

[/追記]